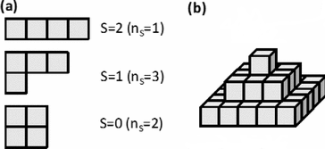
Irreducible representations of the SU(4) group for efficient simulations of open quantum systems with both collective and single-particle decoherence. This produces a pyramid structure of states that are SU(4) symmetric which allows the density matrix of an open cavity QED system to be block diagonal, drastically reducing the complexity of exact quantum simulations to polynomial scaling.
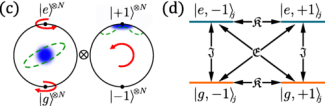
Three-axis twisting in a closed SU(4) system. The cavity interaction not only causes interparticle entanglement in both the spin degree of freedom and momentum degree of freedom, but also entangles the spin and momentum degrees of freedom with one another. Here, the system's dynamics are restricted to two internal states and two momentum states, which can be justified with a Kapitza-Dirac cavity.
Unlike the simple linear scaling of a system of particles in classical mechanics, a system of particles in quantum mechanics scales exponentially which makes direct simulation of anything but the smallest particle numbers impossible. This problem is compounded when considering open quantum systems, as these models are typically described in Liouville space which is a higher dimensional space whose elements are superoperators that act on both sides of a density matrix in order to encapsulate correlations with the environment. However, the symmetric coupling of atoms in cavity QED systems allowed our group to develop a groundbreaking exact quantum simulation of systems with both collective and single-particle decoherence whose numerical complexity is only polynomial-scaled with particle number. This simulation uses the irreducible representations of the SU(4) Lie group to find a permutationally symmetric subspace that forms a pyramid structure, and this pyramid is then used to rewrite the density matrix in a basis in which it is block diagonal with all other elements being zero and decoupled. Our work has been implemented in the Quantum Toolbox in Python (QuTiP) package by researchers from other universities.
This work on utilizing Lie group symmetries to simulate open quantum systems was primarily studied to examine quantum synchronization and superradiance. However, it has also inspired a new avenue in developing quantum sensors by studying entanglement generation schemes in higher dimensions. Such systems can speed up entangling dynamics as well as drastically loosen experimental restrictions that are typically imposed to constrain the dynamics to two quantum levels. Using Lie group symmetries, we develop general polynomial-scaled exact simulations of n-dimensional systems, as well as semi-classical techniques to simulate systems with tens of thousands of particles. In particular, our group is interested in systems where both interparticle and intraparticle entanglement is generated, the latter of which could be between multiple degrees of freedom of a particle such as spin and momentum. We have developed a scheme in which particles undergo three-axis twisting where the ensemble squeezes in both the spin degree of freedom and momentum degree of freedom, but also entangles the spin and momentum degrees of freedom with one another. We have also proposed an SU(4) laser system that has the potential to continuously generate entanglement as a thermal beam passes through a cross-cavity setup as a preparatory stage for metrology. Our work on finding the optimal generator for quantum sensing opens the pathway to unravel the complicated correlations in these higher dimensional systems so that practical sensing applications can be developed.