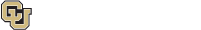Most scientists think it is really hard to correlate, or entangle, the quantum spin states of many particles in an ultracold gas of fermions. Fermions are particles like electrons (and some atoms and molecules) whose quantum spin states prevent them from occupying the same lowest-energy state and forming a Bose-Einstein condensate. Entanglement means that two or more particles interact and retain a connection. Once particles are entangled, if something changes in one of them, all linked partners respond.
Because of this exquisite connection, entanglement is usually destroyed by messing up the state of a single particle. Consequently, conventional wisdom has been that it would take carefully tailored measurements or control schemes to entangle the quantum spin states of hundreds or thousands of atoms in an ultracold gas. However, graduate student Michael Foss-Feig, Fellows James Thompson and Ana Maria Rey, and former Visiting Fellow Andrew Daley from the University of Pittsburgh have just turned this conventional wisdom upside down by considering what happens if the state of pairs of atoms are messed up together.
Foss-Feig and his colleagues have discovered that when reactive fermions are at relatively “warm” micro-Kelvin temperatures, entanglement evolves naturally. In fact, the atomic or molecular gas has to be a 10–100 times warmer than your usual ultracold (nano-Kelvin) gas to encourage entanglement. Once the temperatures have reached the point where fermions collide and react in pairs, atoms or molecules that don’t get knocked out of the experiment will be left entangled because they lose their individual identities as a result of being unable to collide. Fermions that behave this way include the atoms strontium (Sr) and ytterbium (Yb), which are used in atomic clocks, and molecules such as potassium-rubidium (KRb), which are used in JILA cold-molecule experiments.
To understand how this entanglement evolves, imagine that our quantum gas is a tango dancing party. Sr atoms, Yb atoms, or KRb molecules — all of which can exist in one of two possible spin states — are the tango dancers. Just as the atoms or molecules have two possible spin states, there are two kinds of tango dancers: men and women. And, at this quantum dance party, women must dance with men and vice versa. The catch is that the individual tango dancers all dance a little differently.
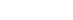
As the dance starts, pairs of tango dancers bump into each other. As they collide, each pair of dancers measures their mutual quantum state to discover whether they dance well together or dance poorly together. When a man and a woman who dance well together find each other, they tango dance their way right out of the party and go home together. Soon, all the men and women who dance well have reacted with each other and gone home.
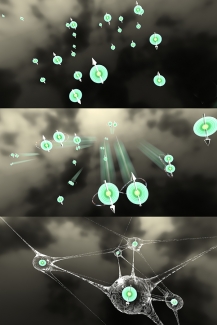
Now the only ones left at the tango dance are the men and women who don’t dance well together. It’s not that these individuals are bad or good tango dancers, though. They don’t dance together because they are correlated with each other in a way that makes it impossible to dance in pairs (i.e., collide). And, because all the remaining tango dancers don’t dance well together, no one can go home. Frustrated (but also slowly losing their individual identities), the party-goers constantly check their watches to see when this boring dance will end. What they don’t realize is that they’re stuck in an unending (steady-state) party where nobody dances, but no one can escape.
The nondancing pairs have entered a quantum mechanical state called a superposition. A superposition is a state in which a particle holds two different properties — such as two different spin states — at the same time. It turns out that such a state is very useful for measuring the passage of time in an atomic clock, for reasons that can be well understood through the connection to dancers.
According to the strange rules of quantum mechanics, the pairs of Sr or Yb atoms or KRb molecules that react (i.e., tango dance well together) are useless for measuring time with an atomic clock. In fact, they are completely oblivious to the passage of time. The pairs that dance poorly together, however, are acutely aware of the passage of time, constantly wondering when the dance will be over. Consequently, a steady-state tango dance party, with everyone constantly checking their watches, could be an ideal starting point for measuring time with an atomic clock. — Julie Phillips and Michael Foss-Feig