The Rules of 4-dimensional Perspective
What does a scene look like when you move through it at near the speed of light?

Accelerate to near the speed of light

Like Hans Solo on the Millenium Falcon, or Captain Picard making it so, you press the hyperlight button, and ... what happens?

In this movie, you accelerate towards the Sun, accelerating at the speed of light per 8 seconds, equivalent to 4 million gees (ouch). You accelerate for 16 seconds, then coast for another 16 seconds. The clock shows your proper time (the time you actually experience) in seconds. The planet is Mercury, not to scale. The real Mercury is smaller and farther from the Sun.

The movie gives the impression that initially you move away from the Sun, but this is false. As you accelerate towards the Sun, relativistic aberration concentrates the scene more and more ahead of you.

Notice several effects, collectively called relativistic beaming: Aberration: the scene is concentrated ahead, expanded behind; Color shifts: the scene is blueshifted ahead, redshifted behind; Brightness changes: the scene is brightened ahead, dimmed behind; Time changes: the scene is speeded up ahead, slowed down behind.

3D Perspective

Leonardo da Vinci learned the rules of 3-dimensional perspective as an apprentice to Andrea del Verrochio in Florence (source: Museum of Science). At left is Leonardo da Vinci's perspective study for "Adoration of the Magi", circa 1481.

In 3-dimensional perspective, two ideas are fundamental: A straight line in 3 dimensions remains a straight line in perspective; Parallel lines meet at a vanishing point.

The Rules of 4-Dimensional Perspective

The rules that govern the appearance of a scene when you move through it at near the speed of light can be called “The Rules of 4-Dimensional Perspective”. These rules can be grasped, much like the rules of 3-dimensional perspective, without needing to understand intricate mathematics.

The Rules of 4-Dimensional Perspective can be summarized as follows: Paint the scene at rest on the surface of a celestial sphere; Stretch the celestial sphere by Lorentz factor γ along the direction of motion into a celestial ellipsoid, and displace the observer to a focus of the ellipsoid; Adjust the brightness, color, and clock speed at any point on the ellipsoid in proportion to the length of the radius between the point and the observer.

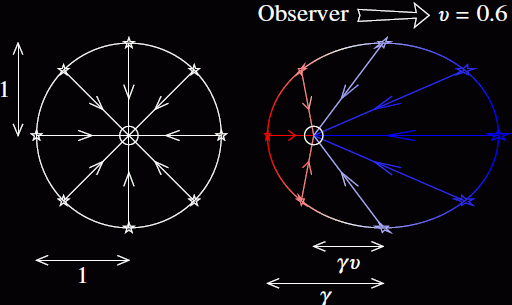
The diagram at left encapsulates these Rules. In the left panel of the diagram, you, the observer, are at the center of the celestial sphere. The stars on the celestial sphere represent, well, stars on the celestial sphere. In the right panel of the diagram, you are moving through the scene to the right at 60% of the speed of light. You, the observer, are at a focus of the celestial ellipsoid. The ellipsoid is stretched by Lorentz factor γ = 1/√(1 − 0.6²) = 1.25 along the direction of motion. The scene appears relativistically aberrated, which is to say concentrated ahead of you, and expanded behind you.

The arrowed lines converging on you the observer represent the energy-momenta of photons that you see. The lengths of the arrows are proportional to the energies, or frequencies, of the photons that you see. The lengths are also proportional to the brightness, the number of photons per unit time, that you see. When you are moving through the scene at near light speed, the arrows ahead are longer, so you see the scene ahead is brighter, and photons ahead are blueshifted, increased in energy, increased in frequency. Conversely, the arrows behind you are shorter, so you see scene behind is dimmer, and the photons behind are redshifted, decreased in energy, decreased in frequency. Since photons are good clocks, the change in photon frequency also tells you how fast or slow clocks attached to the scene appear to you to run.

4D Perspective

The image at left shows Leonardo da Vinci's study for the “Adoration of the Magi” seen at 0.995 times the speed of light. Leonardo's drawing is actually behind us here, but relativistic beaming aberrates it into view. The region ahead is empty because Leonardo's drawing does not cover the whole scene.

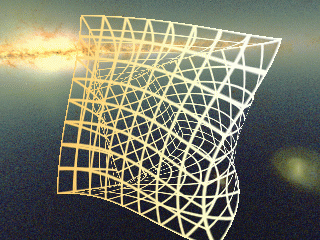
The cutaway image at right illustrates how the relativistic image at left was made. Leonardo's drawing drapes and stretches on to the surface of a celestial ellipsoid, according to the rules of 4D perspective. You, the observer, are at the focus of the ellipsoid close to the left edge of the cutaway image.

In 4-dimensional perspective, straight lines in general do not remain straight: they become arcs of circles. Circles remain circles, although changed in radius.

Relativistically rotating box

This is a relativistically rotating box. The angular velocity is such that corners of the box move at about 1/2 the speed of light.

Despite appearance, the box is not flexing. The box appears to be bent because the light travel time from the far side of the box is longer than that from the near side. You see the far side of the box as it used to be, from a longer time ago than the near side. The difference in light travel times causes approaching parts of the rotating box to appear speeded up, and receding parts of the box to appear slowed down.

Superluminal jets

The giant elliptical galaxy M87 at the center of the Local Supercluster of galaxies has a jet emerging from it. Blobs in the jet are observed to be moving away from the nucleus of the galaxy at about 6 times the speed of light. The observed superluminal (faster-than-light) motion does not violate special relativity, but rather results from relativistic beaming. The jet is actually pointed not sideways, but mostly towards us. Relativistic beaming causes the approaching jet to appear speeded up. The apparent speeding up is a light travel time effect. The light travel time from parts of the jet nearer the galaxy's nucleus, which are farther from us, is longer than the light travel time from parts farther from the nucleus, which are closer to us. The different light travel times give the impression that the jet is moving faster than it really is.

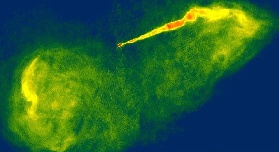
The radio image at right shows that M87 emits twin jets in opposite directions. The two jets extend to near the edge of the visible galaxy, but eventually impact on the tenuous gas of the surrounding intergalactic medium. The collision shocks and slows the jets, pumping twin lobes of relativistic plasma. The radio emission is synchrotron radiation produced by relativistic electrons spiraling around magnetic fields.

Only the approaching jet is observed in visible light. Relativistic beaming brightens the approaching jet, and dims the receding jet to unobservability. Relativistic beaming also blueshifts the approaching jet and redshifts the receding jet, but it is the brightening and dimming that principally causes the apparent one-sideness. If the motion of the receding jet were visible, it would appear slowed down, just as the approaching jet appears speeded up.