Challenge
Cottoned on?
Then you've understood the crux of special relativity,
and you can now go away and figure out all the mathematics
of Lorentz transformations.
Just like Einstein.
The mathematical problem is:
what is the relation between the spacetime coordinates
\(( t , x , y , z )\)
and
\(( t' , x' , y' , z' )\)
of a spacetime interval, a 4-vector, in Vermilion's versus Cerulean's frames,
if Cerulean is moving relative to Vermilion at velocity \(v\) in,
say, the \(x\) direction?
The solution follows from requiring
-
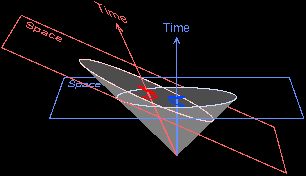
that both observers consider themselves to be at the centre of the lightcone,
and
-
that distances perpendicular to the direction of motion remain unchanged,
as illustrated above.
[An alternative version of the second condition
is that a Lorentz transformation at velocity \(v\) followed
by a Lorentz transformation at velocity −\(v\) should yield
the unit transformation.
This is illustrated in the Lorentz transformation movie above
(go back up to
Cerulean's point of view),
where the transformation by negative velocity −\(v\) is
accomplished by rotating space by 180°
and then transforming by velocity \(v\).]
Note that the postulate of the existence of globally inertial frames
implies that Lorentz transformations are linear,
that straight lines (4-vectors) in one inertial spacetime frame
transform into straight lines in other inertial frames.
A solution to this problem is given in the next section but two,
Construction of the Lorentz Transformation.
As a prelude,
the next two sections discuss
Simultaneity in Special Relativity
and
Time Dilation.
|
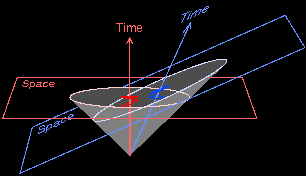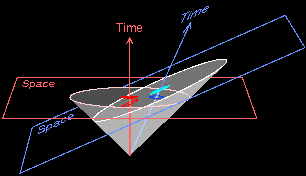 Einstein's solution to the paradox is that
Cerulean's spacetime is skewed compared to Vermilion's.
The thing to notice in the diagram is that Cerulean is in the centre
of the lightcone, according to the way Cerulean perceives space and time.
Vermilion remains at the centre of the lightcone
according to the way Vermilion perceives space and time.
In the diagram
Vermilion
Einstein's solution to the paradox is that
Cerulean's spacetime is skewed compared to Vermilion's.
The thing to notice in the diagram is that Cerulean is in the centre
of the lightcone, according to the way Cerulean perceives space and time.
Vermilion remains at the centre of the lightcone
according to the way Vermilion perceives space and time.
In the diagram
Vermilion